TABLE OF CONTENTS
- A quandary over preserving the “absoluteness” of any observed quantum event
Alice & Bob et al. measure quantum states & wonder about predicting outcomes.
- Visualization of quantum fields (quantum field theory & topology)
In continuous quantum fields, localized disturbances (excitations) interact and exchange energy between fields – as real outcomes.
- A quandary over properties of excitations (wiggles, wave pulses / packets) and superpositions
“We suppress dimensions in order to make sense of things.”
- An unsettling quantum vacuum
Modeling interactions of excitations with vacuum states of quantum fields gets weird.
1. A quandary over preserving the “absoluteness” of any observed quantum event
Here’s a rundown of the “the basic arcana of quantum foundations” and the so-called “measurement problem” in quantum physics. A provocative title at least. Using a coin toss metaphor. No visualizations.
But this article does mention superpositions and wave functions. And so-called “collapse” theories. Information theory. The “many worlds” interpretation (as a “perspectival” theory).
And Alice & Bob (each in their own lab) plus Charlie & Daniela viewing Alice & Bob from the “outside.” (I’m not sure how time is correlated in such a system: “Charlie, for example, treats Alice, her lab and the measurement she makes as one [macroscopic] system that is subject to deterministic, reversible evolution.”)
And spacetime relativity.
So, there’s the question of the “absoluteness of observed events” – whether quantum measurements (of something specific in a specific way for a specific quantum system) are the same for everyone.
Does the discussion conflate “observer” (introducing the notion of choice) with “measurement”? As noted elsewhere, “the universe is always looking,” all the time [1] and outcomes agree just fine. But the discussion here is about OUR ability to predict outcomes.
Any progress in perspective? Or rethink? I still prefer using the phrase wave function “update” vs. “collapse” (and follow experiments which explore whether that really is instantaneous).
There’s no relational quantum theory here (the question of quantum properties as relational vs. intrinsic). No consideration of topology – the topology of: quantum fields, quanta, quantum interactions. And no caution that (mathematical) wavefunctions are simplifications of reality anyway – the article does not address the debate over reality of the wave function.
- Scientific American > “Quantum Theory’s ‘Measurement Problem’ May Be a Poison Pill for Objective Reality” by Anil Ananthaswamy [2] (May 22, 2023) – Solving a notorious quantum quandary could require abandoning some of science’s most cherished assumptions about the physical world.
[Image caption] A core mystery of quantum physics hints that objective reality is illusory – or that the quantum world is even weirder than expected.
At the heart of this bizarreness is what’s called the measurement problem. Standard quantum mechanics accounts for what happens when you measure a quantum system: essentially, the measurement causes the system’s multiple possible states to randomly “collapse” into one definite state. But this accounting doesn’t define what constitutes a measurement – hence, the measurement problem.
In a recent preprint, the trio [Ormrod, Venkatesh, and Barrett] proved a theorem that shows why certain theories – such as quantum mechanics – have a measurement problem in the first place and how one might develop alternative theories to sidestep it, thus preserving the “absoluteness” of any observed event.
[Nicholas Ormrod, University of Oxford] Ormrod says. “If we ever can recover absoluteness, then we’re going to have to give up on some physical principle that we really care about. … Holding on to absoluteness of observed events, it turns out, could mean that the quantum world is even weirder [nuanced] than we know it to be.
…
The article recaps three important properties of perspectival theories, and concludes that “all BIL theories have a measurement problem.”
- Bell nonlocality (B) – entangled, correlated “particle” pairs (as a single inseparable state; so, please avoid the term “linked,” eh).
- Preservation of information (I) – via wave function determinism [while coherent].
- Local dynamics (L) – no faking Bell nonlocality [inter-regional causal affects bound by the speed of light].
Dynamical separability is “kind of an assumption of reductionism,” Ormrod says. … it may be that the dynamics of a [coherent] system are similarly holistic [relational], adding another kind of nonlocality to the universe.
The problem is that no one yet knows how to construct a theory that rejects dynamical separability – assuming it’s even possible to construct – while holding on to the other properties such as preservation of information and Bell nonlocality.
In the end, only experiments will point the way toward the correct theory, …
2. Visualization of quantum fields (quantum field theory & topology)
Visualizing quantum fields is hard. I applaud those who take up the challenge. Here’s Nick Lucid’s latest one.
- YouTube > The Science Asylum > “What Are Particles? Do They ACTUALLY Exist?!” by Nick Lucid (May 1, 2023) – Somewhere between 1926 and 1950, we gave up the concept of particles in favor of quantum fields. In this video, I explain the motivations for that to a non-physicist: my wife.
Time codes:
05:33 What is a Quantum Field?
07:50 Quantum Fields Visualized
… the purpose of quantum field theory. It is to predict how these [so-called] particles will interact. It’s about particle interactions. If there are no particle interactions, quantum field theory is kind of useless.
Every point in space has an existence value, essentially.
And you can see that each of those points are wiggling. Every point is wiggling. Sure. That’s sort of the quantum uncertainty going on here, right?
If there is a large enough disturbance, a full disturbance in this field, we perceive that disturbance as a particle. Okay?
There would be a disturbance at an individual point in a continuous space. Okay. … What you see is a spike in a surface. Which might be called a wave, if you will.
Yes, we would treat the spike that moves around as a wave pulse.
It’s just a disturbance in the electron field, for example. In this case, electric charge is represented by color: red for positive and purple for negative. And quantum spin orientation is represented by the vertical direction: up for spin up and down for spin down. It’s a representation. They’re not really going up and down.
These fields are actually three dimensional, but if I showed you a three dimensional version of this, your eyes and brain would just be overwhelmed and it wouldn’t be useful.
Something we do in physics is we suppress dimensions in order to make sense of things. It’s very common.
So, visualizations of excitations in fields tend to be oscillations of some type. Perhaps involving higher dimensions. With a confined (point-like) topology but extended in spacetime (so, not mere points).
And interacting within their corresponding field(s) and perhaps the roiling quantum vacuum as well. So, a particular property is relational, inseparable from that dynamic context. Restless. Not fixed. Superposed.
But interactions tune that context, to field contours. As, for example, with alignment to magnetic fields (long underrated compared to electromagnetic fields in classical physics). Superpositions change.
3. A quandary over properties of excitations (wiggles, wave pulses / packets) and superpositions
- YouTube > The Science Asylum > “What Are Particles? Do They ACTUALLY Exist?!” by Nick Lucid (May 1, 2023) – Somewhere between 1926 and 1950, we gave up the concept of particles in favor of quantum fields.
And it’s not like the fields occupy different places in space. They all occupy all space. Correct.
Yes, they’re all coexisting.
They all coexist and overlap each other [cf. Wilczek’s Grid]. Right.
Which allows particle interactions to happen because then energy can be exchanged between fields [cf. tuning forks metaphor]. If you have an electron and a positron come together at the same spot, what you get is anti-matter annihilation.
Okay. Which is how that works. Sure.
They just cancel each other out. They just cancel each other out because they have opposite values and it essentially turns the quantum field to a zero value or what we would call the vacuum state of the field.
And by doing that, by assigning existence to every point in space, you’re essentially assigning all possible properties for those particles, for those things we perceive as particles into that spot. All the information is there.
Hmm.
RESONANCE
A striking aspect of quantum fields is that feeding enough energy into them – “you can slap a field and make some particles” (Paul Sutter) – always generates (according to the Standard Model) identical so-called particles. For some events, lots and lots of them. Hence, analogies to modal resonances, standing waves (e.g., realized “notes” from plucked musical strings). For the photon field, tiny, low-power laser diodes produce lots of photons.
• Classroom physics > Standing Waves, Oscillators, Resonance Mode Shapes
My 2-1-2022 comment for “A particle by any other name?” discusses resonance:
As waves or field vibrations, quantum particles “are localized, resonant excitations of these fields.” Sort of natural frequencies (resonances) in spacetime energy.
Everyday examples of resonance include musical instruments and wineglasses. Macro-scale phenomenon. Classical physics.
• Wiki > Resonance
Resonance phenomena occur with all types of vibrations or waves: there is mechanical resonance, acoustic resonance, electromagnetic resonance, nuclear magnetic resonance (NMR), electron spin resonance (ESR) and resonance of quantum wave functions.
But resonance underlies atomic and molecular behavior as well. Our existence depends on such quantum interactions – “in such varied settings, from everyday life down to the smallest scales.”
As physicist Paul Sutter said, “you can slap a field and make some particles.”
• “Reality is fields” comment (May 8, 2017)
- Quanta Magazine > “How the Physics of Resonance Shapes Reality” by Ben Brubaker, Contributing Writer (January 26, 2022) – The same phenomenon by which an opera singer can shatter a wineglass also underlies the very existence of subatomic particles.
Almost anytime physicists announce that they’ve discovered a new particle, whether it’s the Higgs boson or the recently bagged double-charm tetraquark, what they’ve actually spotted is a small bump rising from an otherwise smooth curve on a plot. Such a bump is the unmistakable signature of “resonance,” one of the most ubiquitous phenomena in nature.
Electrons bound to atoms are a little like sound waves trapped inside flutes. As for the atomic nuclei, further advances in the 1930s showed that many kinds of atomic nuclei only exist in the universe today because of resonance.
The frequencies at which quantum fields prefer to vibrate stem from fundamental constants whose origins remain obscure; these frequencies in turn determine the masses of the corresponding particles. Blast the vacuum of empty space hard enough at the right frequency, and out will pop a bunch of particles.
In this sense, resonance is responsible for the very existence of particles.
TOPOLOGY
And as to quantum topology? Like for spinors (fermions). Mostly silence on that topic – while we just do the calculations, eh. Any visualization possible? – without overwhelming our “eyes and brain.”
4. An unsettling quantum vacuum

So, how is the quantum vacuum modeled in quantum field theory? As virtual, mathematical interactions … or as real interplay between fields and excitations?
- YouTube > The Science Asylum > “What Are Particles? Do They ACTUALLY Exist?!” by Nick Lucid (May 1, 2023) – Somewhere between 1926 and 1950, we gave up the concept of particles in favor of quantum fields.
And so what the standard model tells us is that different particles, different fields interact in different ways. That make sense. And we do the calculations using things like Feynman diagrams.
Time codes
10:26 Feynman Diagrams Explained
12:20 Virtual Particles Explained
13:50 How to use Feynman Diagrams
15:05 Quantum Electrodynamics
An excellent introduction to Feyman diagrams – “to represent that [particle interactions] and be able to calculate what’s going to happen with quantum fields.”
(from transcript)
The jiggly spots is the vacuum state of the quantum field. What if we can pretend that those wiggles and jiggles are particles? Because if they’re not necessarily zero, but they’re not like a whole occupation of a spot … So they’re not actual particles, but maybe we can pretend they’re particles, something that we now call virtual particles. They’re not actually particles, but we can pretend that they are. If we do that, then we can start to simplify the math a little bit.Something you might have noticed in those diagrams is that there’s really only one type of vertex.
Every single one of these vertexes has two straight lines and a wiggly line. That’s all that can exist in what we call quantum electrodynamics, which is a type of quantum field theory.
So here’s one scenario. You’ve got an electron and a positron coming together, annihilating and making a photon.
But if I turn [rotate the vertex] this [2nd scenario] … Now, we’ve got an electron coming in, an electron going out, and a photon going out. So now we have an electron emitting a photon and changing direction.
Then [3rd scenario] here we have an electron absorbing a photon and then changing direction. But still an electron. But still an electron. Both the arrows are pointed up in time. Yep.
What we have here [4th scenario]? A photon coming in and dividing into an electron and a positron. Exactly. Ya. See? This is how you read these diagrams.
WHAT QUANTUM FIELD THEORY SAYS ABOUT THE REAL WORLD
His failure, Feynman admitted, was to understand what the math was saying. It provided numbers: predictions of quantities that could be tested against experiments, and which invariably survived those tests. But Feynman couldn’t figure out what these numbers and equations were really about: what they said about the ‘real world’. — Ball, Philip. Beyond Weird (pp. 6-7). University of Chicago Press. Kindle Edition.
Notes
[1] Re “the universe is always looking,” see, for example, Quotes > Philip Ball.
And measurements (which do not require any observer) are about interactions, quantized transactions of energy.
[2] Anil Ananthaswamy is author of The Edge of Physics (Houghton Mifflin Harcourt, 2010), The Man Who Wasn’t There (Dutton, 2015), and Through Two Doors at Once: The Elegant Experiment That Captures the Enigma of Our Quantum Reality (Dutton, 2018).
Recent Articles by Anil Ananthaswamy
- Is Our Universe a Hologram? Physicists Debate Famous Idea on Its 25th Anniversary
- Astronomers Might See Dark Matter by Staring into the Void
- Astronomers Gear Up to Grapple with the High-Tension Cosmos
Related posts (including comments)
- Quantum reality, quantum worlds – new book explores quantum foundations
- 2022 Nobel Prize – ‘spooky action’ pioneers
- A particle by any other name?
- QFT – fields and wave packets
- Feynman’s legacy — quantum originality

So, you can learn to read Feynman diagrams. But how do you setup the path integral sum? – which paths should you sum.
• Wired > “How Reality May Be a Sum of All Possible Realities” by Charlie Wood (Apr 30, 2023) [reprinted with permission from Quanta Magazine] – Richard Feynman’s path integral is a powerful prediction machine and a philosophy. Physicists still struggle to figure out how to use it, and what it means.
A gravitational path integral? – with time or timeless.
Credit: Caltech Weekly 6-28-2022
Speaking of the formulation of path integrals (which paths should you sum), here’s an abstract of a research paper on this problem.
Quantum wave functions can be measured? Directly? And the density matrix.
There are 31 references on quantum mechanics (including a 1948 paper by Feynman): wave functions, (quantum-dot) single-photon sources & interference, (entangled) optical photon pairs, (average) trajectories of single photons, strong & weak measurements, spin (polarization state) measurement, and the principle of least action.
Wiki articles discuss some of the terminology and concepts [1][2][3].
• Nature.com > “Demonstration of the quantum principle of least action with single photons” by Yong-Li Wen et al. (May 22, 2023) [Full article requires a subscription.]
Notes
[1] Here’s an interesting section from the Wiki article on Path Integral Formulation (the article references renormalization, a limiting procedure to treat infinities).
[2] Ibid. there’s a section on the propagator.
[3] Wiki > Propagator
…
(Wiki image as is)
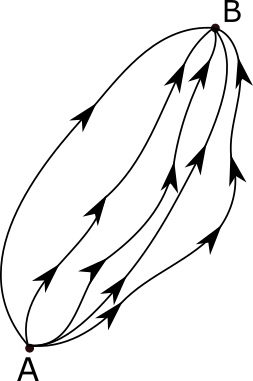
Image licensed under the Creative Commons Attribution-Share Alike 4.0 International license.
The quest for the quantum signature of Planck scale spacetime.
Here’s another article on research which uses the path integral formulation (“the quantum ingredient”). Using computer simulations (numerical methods) for fabrics of spacetime. A most exotic approach to quantum gravity – a theory known as causal dynamical triangulations (CDT) – as to how the spacetime fabric itself evolves.
“The detector makes a click, and that’s a photon (a local bit of energy). But does the graviton … exist in the same way?”
• Quanta Magazine > “The Physicist Who Glues Together Universes” by Charlie Wood (May 25, 2023) – Renate Loll has helped pioneer a radically new approach to quantum gravity. She assumes that the fabric of space-time is a blend of all possible fabrics, and she has developed the computational tools needed to calculate the far-reaching implications of that assumption.